Explore curiosidades, brilhe nos desafios e concorra a prêmios de verdade!
 O poema “Jabberwocky” é o mais famoso poema nonsense de Lewis Carroll, presente em Através do Espelho e o que Alice encontrou lá, 1871. Mistura palavras inventadas com sonoridade poética.
O poema “Jabberwocky” é o mais famoso poema nonsense de Lewis Carroll, presente em Através do Espelho e o que Alice encontrou lá, 1871. Mistura palavras inventadas com sonoridade poética.Jaguadarte
Era briluz. As lesmolisas touvas
roldavam e relviam nos gramilvos;
estavam mimsicais as pintalouvas,
e os momirratos davam grilvos.
“Cuidado! O Jaguadarte, meu filho!
as garras que agarram, a bocarra que morde!
Cuidado com o pássaro Júbju!
Fuja do frumioso Bandersnorte!”
Ele arrancou a espada vorpalina:
foi atrás do inimigo manxome.
Junto ao Tumtumeiro descansou,
e ficou cismando, em plúmbea meditação.
E no ufano pensamento eis que,
com olhos de fogo, o Jaguadarte
veio fuzilando pelo bosque tulgi,
e roncava enquanto vinha!
Um, dois! Um, dois! E a espada vorpalina
vai-vem, vem-vai, para cá e para lá!
Trinchou o monstro e, finda a peleja,
com a cabeça triunfante voltou galumphando.
“Matou o Jaguadarte? Abraça-me,
menino luzidio! Frabjoso dia!
Callooh! Callay!” — ele riu em seu júbilo.
Era briluz. As lesmolisas touvas
roldavam e relviam nos gramilvos;
estavam mimsicais as pintalouvas,
e os momirratos davam grilvos.
 O clássico de terror Poltergeist (1982), dirigido por Tobe Hooper e produzido por Steven Spielberg, ficou famoso não apenas por sua história assustadora, mas também pela chamada “maldição de Poltergeist”. Ao longo dos anos, diversos membros do elenco e da equipe técnica morreram de forma precoce e, muitas vezes, misteriosa, alimentando lendas em torno da produção.
O clássico de terror Poltergeist (1982), dirigido por Tobe Hooper e produzido por Steven Spielberg, ficou famoso não apenas por sua história assustadora, mas também pela chamada “maldição de Poltergeist”. Ao longo dos anos, diversos membros do elenco e da equipe técnica morreram de forma precoce e, muitas vezes, misteriosa, alimentando lendas em torno da produção.Heather O’Rourke, que interpretou a pequena Carol Anne, faleceu aos 12 anos devido a complicações médicas inesperadas, enquanto Dominique Dunne, que viveu Dana, foi assassinada por seu ex-namorado pouco após as filmagens. Outros atores e pessoas ligadas ao filme também sofreram mortes prematuras, muitas delas atribuídas a acidentes ou doenças súbitas, reforçando a aura de mistério que envolve a obra.
Especialistas e fãs de filmes de terror apontam que essas tragédias, somadas aos eventos paranormais retratados no longa, contribuíram para que Poltergeist se tornasse uma lenda urbana no mundo cinematográfico. A combinação de terror na tela e acontecimentos fora dela transformou o filme em um ícone do gênero, provocando fascínio e medo décadas depois de seu lançamento.
 Os desertos são formações naturais caracterizadas por baixos índices pluviométricos (geralmente abaixo de 250 mm/ano), altas amplitudes térmicas e vegetação escassa. No entanto, apesar de parecerem espaços inóspitos e estáticos, eles também são fruto de processos de produção do espaço geográfico, ou seja, de relações entre a sociedade e a natureza.
Os desertos são formações naturais caracterizadas por baixos índices pluviométricos (geralmente abaixo de 250 mm/ano), altas amplitudes térmicas e vegetação escassa. No entanto, apesar de parecerem espaços inóspitos e estáticos, eles também são fruto de processos de produção do espaço geográfico, ou seja, de relações entre a sociedade e a natureza.A produção do espaço nos desertos envolve práticas como a agricultura irrigada, a mineração, a urbanização e o turismo. Por exemplo, no deserto do Saara, há extração de petróleo e fosfato; no Oriente Médio, o uso intensivo da irrigação com tecnologia avançada transformou áreas áridas em zonas agrícolas. Já nos Estados Unidos, especialmente no deserto de Nevada, surgem cidades como Las Vegas, resultado de fortes investimentos em infraestrutura e energia.
Essas transformações exigem tecnologias específicas, como dessalinização, irrigação por gotejamento e uso de energia solar. Ao mesmo tempo, tais atividades podem gerar impactos ambientais, como o esgotamento de aquíferos e a salinização dos solos.
Portanto, os desertos são também espaços geográficos em constante transformação, moldados pelas necessidades humanas, pelas políticas de uso do solo e pelos avanços tecnológicos, revelando a complexa interação entre sociedade e meio ambiente.
 Tudo começou com Adão e Eva, o primeiro homem e a primeira mulher criados por Deus. Eles viviam no Jardim do Éden, um lugar lindo e perfeito. Mas um dia, desobedeceram a Deus e comeram o fruto proibido. Isso nos ensina a importância de obedecer a Deus e fazer o que é certo.
Tudo começou com Adão e Eva, o primeiro homem e a primeira mulher criados por Deus. Eles viviam no Jardim do Éden, um lugar lindo e perfeito. Mas um dia, desobedeceram a Deus e comeram o fruto proibido. Isso nos ensina a importância de obedecer a Deus e fazer o que é certo.Depois, temos a história emocionante de Moisés! Ele foi escolhido por Deus para libertar o povo de Israel da escravidão no Egito. Com a ajuda de Deus, Moisés abriu o Mar Vermelho, e o povo passou por terra seca! Uau! Imagina só ver um mar se abrindo! Essa história mostra como Deus cuida de quem confia nele.
E não podemos esquecer de Davi e Golias. Davi era um menino pastor que enfrentou o gigante Golias com apenas uma pedra e um estilingue. Ele não teve medo porque sabia que Deus estava com ele. E venceu! Essa história mostra que, mesmo pequenos, podemos vencer grandes desafios quando confiamos em Deus.
 9 de Julho é dia da Revolução Constitucionalista de 1932, movimento que marcou a luta dos paulistas por uma nova Constituição para o Brasil. A revolta foi organizada pelo Partido Republicano Paulista (PRP), com apoio do Partido Democrático, e contou com a participação de civis e militares paulistas, além de apoio dos estados de Mato Grosso e Rio Grande do Sul. O estopim foi a morte de quatro jovens durante um protesto contra o governo de Getúlio Vargas, dando origem ao movimento MMDC. Embora as tropas paulistas tenham sido derrotadas em outubro de 1932, a mobilização resultou na convocação da Assembleia Constituinte de 1933 e na promulgação da Constituição de 1934.O dia 9 de julho foi instituído como feriado estadual em 1997, após aprovação do Projeto de Lei nº 710/1995, e é exclusivo de São Paulo. A data homenageia o esforço por democracia e é lembrada como um marco da história paulista.
9 de Julho é dia da Revolução Constitucionalista de 1932, movimento que marcou a luta dos paulistas por uma nova Constituição para o Brasil. A revolta foi organizada pelo Partido Republicano Paulista (PRP), com apoio do Partido Democrático, e contou com a participação de civis e militares paulistas, além de apoio dos estados de Mato Grosso e Rio Grande do Sul. O estopim foi a morte de quatro jovens durante um protesto contra o governo de Getúlio Vargas, dando origem ao movimento MMDC. Embora as tropas paulistas tenham sido derrotadas em outubro de 1932, a mobilização resultou na convocação da Assembleia Constituinte de 1933 e na promulgação da Constituição de 1934.O dia 9 de julho foi instituído como feriado estadual em 1997, após aprovação do Projeto de Lei nº 710/1995, e é exclusivo de São Paulo. A data homenageia o esforço por democracia e é lembrada como um marco da história paulista. Halloween
Halloween Harry Potter
Harry Potter Acadêmico
Acadêmico Stranger Things
Stranger Things Esportes
Esportes The Walking Dead
The Walking Dead Cervejas
Cervejas Música
Música Games
Games Copa Libertadores
Copa Libertadores Cinema e TV
Cinema e TV Turismo
Turismo Copa Futebol Feminino
Copa Futebol Feminino Samba & Pagode
Samba & Pagode KPOP
KPOP Animações
Animações Astrologia
Astrologia Surf
Surf MPB
MPB Jogos de tabuleiro
Jogos de tabuleiro MineCraft
MineCraft Cinema
Cinema Ginástica
Ginástica FortNite
FortNite Jogos de carta
Jogos de carta Funk
Funk Novelas brasileiras
Novelas brasileiras Hip Hop
Hip Hop Futebol
Futebol Turismo Europa
Turismo Europa RPG
RPG Jogos eletrônicos
Jogos eletrônicos Basquetebol
Basquetebol Barbie
Barbie Matemática
Matemática Rock n Roll
Rock n Roll Ciências Naturais
Ciências Naturais MarioBros
MarioBros Língua Inglesa
Língua Inglesa Atletismo
Atletismo Roblox
Roblox Voleibol
Voleibol Folclore Português
Folclore Português Música Pop
Música Pop Portugal
Portugal Sertanejo
Sertanejo Natação
Natação Séries de TV
Séries de TV Físico-Química
Físico-Química Olimpiadas
Olimpiadas Forró
Forró Tênis
Tênis História
História Língua Portuguesa
Língua Portuguesa Turismo América do Norte
Turismo América do Norte Turismo América do Sul
Turismo América do Sul Geografia
Geografia Turismo no Brasil
Turismo no Brasil Magic the Gathering
Magic the Gathering Turismo na França
Turismo na França Turismo no Chile
Turismo no Chile Vinhos Portugueses
Vinhos Portugueses Turismo em Portugal
Turismo em Portugal Brasileirão
Brasileirão Turismo na Espanha
Turismo na Espanha Yoga
Yoga Futebol Brasileiro
Futebol Brasileiro Turismo no Canadá
Turismo no Canadá Anime
Anime Turismo em Bonaire
Turismo em Bonaire Turismo no Reino Unido
Turismo no Reino Unido Turismo em Curaçau
Turismo em Curaçau Turismo na Colombia
Turismo na Colombia Guiana Francesa
Guiana Francesa Turismo no Peru
Turismo no Peru Turismo no Suriname
Turismo no Suriname Turismo no México
Turismo no México TUruguai
TUruguai NBA
NBA Turismo no Paraguai
Turismo no Paraguai Turismo em Guiana
Turismo em Guiana Variedades
Variedades Turismo na Argentina
Turismo na Argentina BlockBusters
BlockBusters Turismo em Aruba
Turismo em Aruba Futebol Espanhol
Futebol Espanhol Turismo Ilhas Malvinas
Turismo Ilhas Malvinas Futebol Português
Futebol Português Turismo Estados Unidos
Turismo Estados Unidos Turismo na Venezuela
Turismo na Venezuela Turismo na Itália
Turismo na Itália Turismo na Bolívia
Turismo na Bolívia Desenhos Animados
Desenhos Animados SL Benfica
SL Benfica Pré-História
Pré-História Idade Antiga
Idade Antiga Marvel
Marvel FESTA de são joão
FESTA de são joãoSabia que hoje é dia de..
 20 de outubro dia de Combate ao Bullying. Esta data foi criada com o objetivo de conscientizar a sociedade sobre a importância de prevenir e combater o bullying, um problema que afeta crianças, adolescentes e até adultos em diversos ambientes, especialmente nas escolas. O bullying é caracterizado por comportamentos agressivos e repetitivos, como intimidações, humilhações e agressões físicas ou verbais, que têm um impacto negativo profundo na saúde mental e emocional das vítimas. A campanha de conscientização busca promover o respeito, a empatia e a inclusão, além de incentivar ações preventivas e educativas que ajudem a criar ambientes seguros e saudáveis para todos.
20 de outubro dia de Combate ao Bullying. Esta data foi criada com o objetivo de conscientizar a sociedade sobre a importância de prevenir e combater o bullying, um problema que afeta crianças, adolescentes e até adultos em diversos ambientes, especialmente nas escolas. O bullying é caracterizado por comportamentos agressivos e repetitivos, como intimidações, humilhações e agressões físicas ou verbais, que têm um impacto negativo profundo na saúde mental e emocional das vítimas. A campanha de conscientização busca promover o respeito, a empatia e a inclusão, além de incentivar ações preventivas e educativas que ajudem a criar ambientes seguros e saudáveis para todos. 20 de outubro dia das Mastocitoses e Patologias Mastocitárias para aumentar a conscientização sobre essas doenças raras, causadas pelo excesso ou ativação excessiva dos mastócitos, células do sistema imunológico. O diagnóstico dessas condições pode ser demorado e difícil, uma vez que os sintomas se assemelham a reações alérgicas e surgem em resposta a fatores como medicamentos, picadas de insetos, alimentos e estresse emocional. As mastocitoses podem ser classificadas em mastocitose cutânea, que afeta a pele, e mastocitose sistêmica, que envolve múltiplos sistemas do corpo. Os sintomas variam, incluindo reações alérgicas, urticária, coceira e dor abdominal. O tratamento envolve a evitação de gatilhos específicos e a prescrição de medicamentos que estabilizam os mastócitos.
20 de outubro dia das Mastocitoses e Patologias Mastocitárias para aumentar a conscientização sobre essas doenças raras, causadas pelo excesso ou ativação excessiva dos mastócitos, células do sistema imunológico. O diagnóstico dessas condições pode ser demorado e difícil, uma vez que os sintomas se assemelham a reações alérgicas e surgem em resposta a fatores como medicamentos, picadas de insetos, alimentos e estresse emocional. As mastocitoses podem ser classificadas em mastocitose cutânea, que afeta a pele, e mastocitose sistêmica, que envolve múltiplos sistemas do corpo. Os sintomas variam, incluindo reações alérgicas, urticária, coceira e dor abdominal. O tratamento envolve a evitação de gatilhos específicos e a prescrição de medicamentos que estabilizam os mastócitos. 20 de outubro dia do Controlador de Tráfego Aéreo, para homenagear esses profissionais e reconhecer a importância de seu trabalho na segurança da aviação civil. A data valoriza os controladores que gerenciam o tráfego aéreo, assegurando que as aeronaves decolam e pousam com segurança. O objetivo do dia é aumentar a conscientização sobre os desafios enfrentados no controle de tráfego aéreo, fundamental para a segurança e eficiência das operações. Além disso, busca promover melhorias nas condições de trabalho e investimentos em tecnologia e formação. Os controladores de tráfego aéreo têm um papel crucial na coordenação das aeronaves nos aeroportos e no espaço aéreo, evitando colisões e garantindo rotas seguras.
20 de outubro dia do Controlador de Tráfego Aéreo, para homenagear esses profissionais e reconhecer a importância de seu trabalho na segurança da aviação civil. A data valoriza os controladores que gerenciam o tráfego aéreo, assegurando que as aeronaves decolam e pousam com segurança. O objetivo do dia é aumentar a conscientização sobre os desafios enfrentados no controle de tráfego aéreo, fundamental para a segurança e eficiência das operações. Além disso, busca promover melhorias nas condições de trabalho e investimentos em tecnologia e formação. Os controladores de tráfego aéreo têm um papel crucial na coordenação das aeronaves nos aeroportos e no espaço aéreo, evitando colisões e garantindo rotas seguras. 20 de outubro dia da Paralisia Cerebral em Portugal. Foi instituído para aumentar a conscientização sobre essa condição neurológica, que afeta o movimento e a coordenação motora devido a lesões no cérebro durante o desenvolvimento, geralmente antes do nascimento. A data foi comemorada pela primeira vez em Portugal em 2013 e oficializada por decreto-lei em 2014. Seu objetivo é desmistificar preconceitos sobre a paralisia cerebral (PC) e conscientizar a comunidade sobre os desafios enfrentados por pessoas com PC e suas famílias. A paralisia cerebral é a condição motora mais comum em crianças, afetando aproximadamente 2 em cada 1.000 nascimentos.
20 de outubro dia da Paralisia Cerebral em Portugal. Foi instituído para aumentar a conscientização sobre essa condição neurológica, que afeta o movimento e a coordenação motora devido a lesões no cérebro durante o desenvolvimento, geralmente antes do nascimento. A data foi comemorada pela primeira vez em Portugal em 2013 e oficializada por decreto-lei em 2014. Seu objetivo é desmistificar preconceitos sobre a paralisia cerebral (PC) e conscientizar a comunidade sobre os desafios enfrentados por pessoas com PC e suas famílias. A paralisia cerebral é a condição motora mais comum em crianças, afetando aproximadamente 2 em cada 1.000 nascimentos. 20 de outubro dia da Estatística, para ressaltar a importância da estatística em diversas áreas, como ciência, economia e saúde. Os principais objetivos dessa data são: Promover a conscientização sobre a coleta e análise de dados para decisões informadas; Celebrar a profissão de estatístico e suas contribuições à sociedade e Incentivar o uso de dados estatísticos em políticas públicas e pesquisas. Oficialmente proclamado pela ONU em 2010, o dia destaca o papel essencial das estatísticas no desenvolvimento econômico e social. Em todo o mundo, são organizados workshops, conferências e eventos educativos para aumentar a compreensão e o uso adequado de dados estatísticos, reconhecendo seu papel na resolução de problemas e na melhoria da qualidade de vida.
20 de outubro dia da Estatística, para ressaltar a importância da estatística em diversas áreas, como ciência, economia e saúde. Os principais objetivos dessa data são: Promover a conscientização sobre a coleta e análise de dados para decisões informadas; Celebrar a profissão de estatístico e suas contribuições à sociedade e Incentivar o uso de dados estatísticos em políticas públicas e pesquisas. Oficialmente proclamado pela ONU em 2010, o dia destaca o papel essencial das estatísticas no desenvolvimento econômico e social. Em todo o mundo, são organizados workshops, conferências e eventos educativos para aumentar a compreensão e o uso adequado de dados estatísticos, reconhecendo seu papel na resolução de problemas e na melhoria da qualidade de vida.
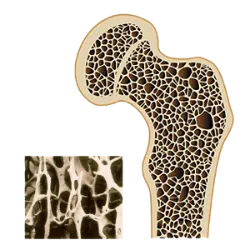 20 de outubro dia da Osteoporose, visa aumentar a conscientização sobre essa condição, que provoca perda de densidade óssea e eleva o risco de fraturas, especialmente entre idosos. Os principais objetivos da data incluem educar o público sobre a osteoporose, promover a detecção precoce por meio de exames de densidade óssea e estimular hábitos saudáveis, como uma dieta rica em cálcio e vitamina D e a prática regular de exercícios. A osteoporose é uma doença crônica que afeta principalmente mulheres pós-menopausa e pode levar a complicações graves, como dores crônicas e redução da qualidade de vida. Fatores de risco incluem idade avançada, histórico familiar, dieta pobre em nutrientes, sedentarismo e deficiências hormonais.
20 de outubro dia da Osteoporose, visa aumentar a conscientização sobre essa condição, que provoca perda de densidade óssea e eleva o risco de fraturas, especialmente entre idosos. Os principais objetivos da data incluem educar o público sobre a osteoporose, promover a detecção precoce por meio de exames de densidade óssea e estimular hábitos saudáveis, como uma dieta rica em cálcio e vitamina D e a prática regular de exercícios. A osteoporose é uma doença crônica que afeta principalmente mulheres pós-menopausa e pode levar a complicações graves, como dores crônicas e redução da qualidade de vida. Fatores de risco incluem idade avançada, histórico familiar, dieta pobre em nutrientes, sedentarismo e deficiências hormonais.
 20 de outubro dia do Arquivista no Brasil. Esta data homenageia os profissionais responsáveis por organizar, conservar e disponibilizar documentos e informações, garantindo a gestão adequada de arquivos em diversas instituições, como bibliotecas, empresas, órgãos governamentais e históricos. O papel do arquivista é fundamental para a preservação da memória, da história e para o bom funcionamento administrativo de qualquer organização. Esses profissionais asseguram que os documentos importantes, físicos e digitais, sejam armazenados de forma eficiente e segura, facilitando o acesso futuro. A data foi escolhida em homenagem à fundação da Associação dos Arquivistas Brasileiros (AAB), ocorrida em 20 de outubro de 1971.
20 de outubro dia do Arquivista no Brasil. Esta data homenageia os profissionais responsáveis por organizar, conservar e disponibilizar documentos e informações, garantindo a gestão adequada de arquivos em diversas instituições, como bibliotecas, empresas, órgãos governamentais e históricos. O papel do arquivista é fundamental para a preservação da memória, da história e para o bom funcionamento administrativo de qualquer organização. Esses profissionais asseguram que os documentos importantes, físicos e digitais, sejam armazenados de forma eficiente e segura, facilitando o acesso futuro. A data foi escolhida em homenagem à fundação da Associação dos Arquivistas Brasileiros (AAB), ocorrida em 20 de outubro de 1971. 20 de outubro dia do Maquinista, homenageia os profissionais que conduzem trens e locomotivas, essenciais para o transporte de mercadorias e pessoas no Brasil. Sua função é vital para a segurança e eficiência do transporte ferroviário, exigindo responsabilidade, concentração e conhecimento técnico. A data também reconhece a importância histórica da profissão no desenvolvimento econômico do país, especialmente no transporte de produtos agrícolas e industriais. A escolha do dia se deve à fundação da Associação dos Maquinistas e Ferroviários de São Paulo (AMAFER), em 1907, destacando a longa tradição dessa profissão.
20 de outubro dia do Maquinista, homenageia os profissionais que conduzem trens e locomotivas, essenciais para o transporte de mercadorias e pessoas no Brasil. Sua função é vital para a segurança e eficiência do transporte ferroviário, exigindo responsabilidade, concentração e conhecimento técnico. A data também reconhece a importância histórica da profissão no desenvolvimento econômico do país, especialmente no transporte de produtos agrícolas e industriais. A escolha do dia se deve à fundação da Associação dos Maquinistas e Ferroviários de São Paulo (AMAFER), em 1907, destacando a longa tradição dessa profissão. 20 de outubro dia do Operador de Caixa no Brasil.Esta data foi criada para reconhecer e valorizar o trabalho dos operadores de caixa, profissionais que desempenham um papel fundamental no comércio, especialmente em supermercados, lojas de varejo e estabelecimentos de serviços. Os operadores de caixa são responsáveis por diversas funções, como registrar as compras dos clientes, manusear dinheiro e meios de pagamento, emitir notas fiscais e proporcionar um bom atendimento ao consumidor. Eles atuam na linha de frente das operações comerciais e são essenciais para garantir a satisfação dos clientes, uma vez que representam a última interação do consumidor com a empresa durante uma compra.
20 de outubro dia do Operador de Caixa no Brasil.Esta data foi criada para reconhecer e valorizar o trabalho dos operadores de caixa, profissionais que desempenham um papel fundamental no comércio, especialmente em supermercados, lojas de varejo e estabelecimentos de serviços. Os operadores de caixa são responsáveis por diversas funções, como registrar as compras dos clientes, manusear dinheiro e meios de pagamento, emitir notas fiscais e proporcionar um bom atendimento ao consumidor. Eles atuam na linha de frente das operações comerciais e são essenciais para garantir a satisfação dos clientes, uma vez que representam a última interação do consumidor com a empresa durante uma compra. 20 de outubro dia do Guarda Noturno no Brasil. Esta data foi criada para reconhecer e valorizar o trabalho dos guardas noturnos, que desempenham um papel essencial na segurança de comunidades e estabelecimentos comerciais durante a noite. Os guardas noturnos são responsáveis pela proteção de propriedades e pessoas, atuando como vigilantes em áreas residenciais, comerciais e industriais. Suas funções incluem monitorar o ambiente, fazer rondas, garantir a segurança de bens e pessoas e agir em situações de emergência. Eles muitas vezes trabalham em colaboração com as forças policiais e podem ser a primeira linha de defesa contra crimes e incidentes.
20 de outubro dia do Guarda Noturno no Brasil. Esta data foi criada para reconhecer e valorizar o trabalho dos guardas noturnos, que desempenham um papel essencial na segurança de comunidades e estabelecimentos comerciais durante a noite. Os guardas noturnos são responsáveis pela proteção de propriedades e pessoas, atuando como vigilantes em áreas residenciais, comerciais e industriais. Suas funções incluem monitorar o ambiente, fazer rondas, garantir a segurança de bens e pessoas e agir em situações de emergência. Eles muitas vezes trabalham em colaboração com as forças policiais e podem ser a primeira linha de defesa contra crimes e incidentes. O Dia do Poeta é celebrado em 20 de outubro no Brasil, em homenagem aos escritores que usam criatividade, imaginação e sensibilidade para criar poesias. A data foi escolhida por lembrar a fundação do Movimento Poético Nacional em 1976, na casa do jornalista e escritor Paulo Menotti Del Picchia, em São Paulo. Embora não seja oficializada por lei, é amplamente comemorada no Brasil por escolas e instituições literárias. Além disso, outra data importante para a poesia no Brasil é 14 de março, o Dia Nacional da Poesia, em homenagem ao nascimento do poeta Castro Alves. Já o Dia Mundial da Poesia, instituído pela UNESCO, é celebrado em 21 de março, incentivando a leitura e criação poética em todo o mundo.
O Dia do Poeta é celebrado em 20 de outubro no Brasil, em homenagem aos escritores que usam criatividade, imaginação e sensibilidade para criar poesias. A data foi escolhida por lembrar a fundação do Movimento Poético Nacional em 1976, na casa do jornalista e escritor Paulo Menotti Del Picchia, em São Paulo. Embora não seja oficializada por lei, é amplamente comemorada no Brasil por escolas e instituições literárias. Além disso, outra data importante para a poesia no Brasil é 14 de março, o Dia Nacional da Poesia, em homenagem ao nascimento do poeta Castro Alves. Já o Dia Mundial da Poesia, instituído pela UNESCO, é celebrado em 21 de março, incentivando a leitura e criação poética em todo o mundo.Confira outras curiosidades e desafios de quiz
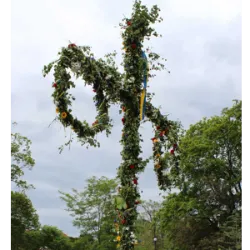
As festas juninas na Suécia, conhecidas como Midsommarafton, são consideradas as celebrações nacionais mais importantes do país, superando até o Natal em participação popular. Realizadas entre 20 e 26 de junho, a sexta-feira é o dia tradicional para a comemoração, marcada por diversas tradições típicas.
Um dos símbolos centrais da festa é o majstången — um mastro decorado com flores e folhas, erguido no centro das vilas, em torno do qual os participantes dançam em círculo. Essa tradição tem raízes comuns ao mastro de maio dos povos germânicos e está ligada à fertilidade e à renovação da natureza.
Durante o Midsommarafton, as pessoas se vestem com roupas rurais, cantam cânticos tradicionais e celebram com comidas típicas da estação, como morangos e batatas. Simpatias também fazem parte da festa, especialmente entre as moças, que confeccionam buquês com sete ou nove flores para sonhar com seus futuros maridos.
Além disso, a crença popular diz que as ervas colhidas nessa época possuem poderes especiais e que a água das fontes traz saúde. Casas são decoradas com flores e folhas para atrair sorte. Grandes cidades como Estocolmo e Gotemburgo ficam quase vazias, pois os suecos se refugiam nas casas de veraneio para celebrar a data. Os tradicionais balões da festa, porém, costumam causar acidentes frequentes.

A grande novidade para a edição de 2025 da Copa do Mundo de Clubes será a presença do Inter Miami CF, clube dos Estados Unidos, que garantiu uma vaga como representante do país-sede. O time norte-americano, que ganhou notoriedade com a chegada do astro Lionel Messi em 2023, conquistou sua classificação ao vencer o MLS Supporters' Shield de 2024, prêmio dado ao time com a melhor campanha na temporada regular da Major League Soccer (MLS).
Essa vaga, inédita para o clube e exclusiva para a equipe do país anfitrião, marca uma importante participação para o futebol dos Estados Unidos, que está em ascensão com o aumento do interesse por grandes estrelas internacionais e o crescimento das competições domésticas. Além disso, a presença do Inter Miami CF no torneio coloca o futebol norte-americano no centro das atenções, atraindo ainda mais público e patrocinadores para o esporte no país.
A classificação do Inter Miami também representa a importância de um campeonato competitivo como a MLS, que começa a se consolidar como uma liga relevante no cenário global. O clube agora terá a chance de enfrentar alguns dos melhores times do mundo no Mundial de Clubes 2025, com a expectativa de deixar sua marca na história do torneio.

Para escolher a cerveja perfeita, experimente diferentes estilos e marcas, leve em conta a harmonização com alimentos e considere sabores, acidez e teor alcoólico. Por exemplo, para massas, escolha Dark Lager, Strong Ale ou Pilsner, enquanto para churrasco, opte por Pilsner, Stout ou Brown Ale. Frutos do mar combinam bem com English Pale Ale, Amber Ale, Hefeweizen e Witbier, e para sobremesas, escolha Stout, Porter ou Fruit Lambic. Queijos podem ser acompanhados por diversas cervejas, mas as escuras como Porter e Stout geralmente funcionam bem. Se for comida vegetariana, a Pilsner é uma boa escolha devido à variedade de ingredientes vegetarianos.
No blog do Syndicato, você encontra mais dicas sobre combinações ideais.

Inaugurado em 1978, o Zoológico Municipal de Volta Redonda se consolidou como um dos maiores e mais importantes espaços de preservação ambiental e lazer do estado do Rio de Janeiro. Localizado no bairro Vila Santa Cecília, o local foi criado com o objetivo de proporcionar contato com a natureza em meio a uma cidade industrial, oferecendo educação ambiental à população e protegendo a fauna silvestre.
Com mais de 300 animais de diferentes espécies — entre aves, mamíferos e répteis — o zoológico se tornou referência regional em cuidados veterinários e programas de conservação. Entre os destaques estão o viveiro de imersão e os espaços temáticos voltados à educação das crianças sobre a importância da biodiversidade e do meio ambiente.
Além de sua função educativa, o zoológico é um dos pontos turísticos mais visitados da cidade, recebendo milhares de visitantes por ano. O espaço conta com trilhas, áreas para piqueniques e parquinhos, integrando lazer e consciência ambiental.
Ao longo dos anos, o Zoológico de Volta Redonda vem passando por reformas e melhorias estruturais, reafirmando seu papel não só como destino turístico, mas também como símbolo da relação entre desenvolvimento urbano e sustentabilidade ambiental.

Vila Nova de Paiva é um verdadeiro centro de tradição artesanal, onde técnicas antigas se mantêm vivas e adaptadas ao tempo. A cultura local é marcada por um rico legado de saberes transmitidos de geração em geração, com destaque para:
A confecção de tecidos em linho, outrora um pilar da economia local, foi sendo substituída pela indústria do algodão, mas ainda se preservam técnicas de produção artesanal, como o burel, tecido em casa, especialmente com a ajuda do pisão de Fráguas, que aprimorava o acabamento. A região também se destaca pela produção de mantas de lã e vestuário de burel, produtos típicos de Vila Nova de Paiva e Pendilhe.
A cerâmica, a azulejaria e o ferro forjado continuam a ser praticados, com destaque para os trabalhos de restauro e esculturas figurativas em granito. A cestaria e a tamancaria são outros ofícios tradicionais de Pendilhe, Alhais, Queiriga e Vila Nova de Paiva, além da produção de miniaturas em madeira de alfaias agrícolas, especialmente em Vila Cova-à-Coelheira.
Os trabalhos de cantaria, escultura em granito e madeira, e a arte das rendas são outras expressões de grande importância na região, mantendo viva a história e a cultura local.

Realismo / Naturalismo / Parnasianismo (século XIX)
Machado de Assis (Dom Casmurro) – Brasil
Eça de Queirós (O Primo Basílio) – Portugal
Gustave Flaubert (Madame Bovary) – França
Lima Barreto (Triste Fim de Policarpo Quaresma) – Brasil
Olavo Bilac – Poesia parnasiana – Brasil
Émile Zola – Naturalismo francês
Modernismo (século XX – até 1945)
James Joyce (Ulisses) – Irlanda
Virginia Woolf (Mrs. Dalloway) – Inglaterra
Franz Kafka (A Metamorfose) – Tchecoslováquia
Mário de Andrade (Macunaíma) – Brasil
Oswald de Andrade (Manifesto Antropofágico) – Brasil
Carlos Drummond de Andrade – Poesia moderna – Brasil
Contemporânea (pós-1945 até hoje)
Clarice Lispector (A Hora da Estrela) – Brasil
Gabriel García Márquez (Cem Anos de Solidão) – Colômbia
José Saramago (Ensaio sobre a Cegueira) – Portugal
Chimamanda Ngozi Adichie – Nigéria, literatura pós-colonial
Haruki Murakami – Japão, surrealismo moderno
Conceição Evaristo – Brasil, literatura afro-brasileira
Margaret Atwood (O Conto da Aia) – Canadá

A
- Normalmente, a ordem em inglês é:
Sujeito + Verbo + Complemento
Exemplo:
Com
-
Exemplos:
-
-
-
-
A inversão acontece às vezes com verbos de movimento:
-
-
-
Em vez de usar if , você pode usar inversão formal:
-

Desde os tempos antigos, os profetas hebreus anunciaram a vinda de um Messias — um enviado de Deus que traria salvação ao Seu povo. Entre essas profecias, uma das mais marcantes está em Isaías 53, onde é descrito um “servo sofredor”, que seria rejeitado, castigado pelas transgressões alheias e, por meio de seu sofrimento, traria cura e paz. Apesar de sua aparente derrota, esse servo seria exaltado por Deus.
Séculos depois, os cristãos veem na vida, morte e ressurreição de Jesus Cristo o cumprimento exato dessas palavras. Crucificado injustamente, Jesus assumiu o sofrimento em favor da humanidade e, ao ressuscitar ao terceiro dia, foi glorificado, confirmando sua identidade como o Messias prometido. Sua ressurreição é, para os fiéis, a prova de que as antigas promessas de Deus se cumpriram de forma plena.
Para os cristãos, Jesus não apenas cumpriu Isaías 53, mas também diversas outras profecias messiânicas espalhadas pelo Antigo Testamento, como em Salmos e Daniel. A Páscoa, portanto, celebra não só um acontecimento histórico, mas a realização de uma esperança profética: o Messias veio, sofreu, morreu e venceu a morte para oferecer salvação eterna a todos os que creem.

1. Estrutura Básica:
Palavra interrogativa + Verbo Auxiliar + Sujeito + Verbo Principal + Complemento?
2. Perguntas com
-
-
3. Perguntas com
-
-
4. Perguntas com
-
-
5. Perguntas com
-
-
Atenção! Se
-
6. Perguntas com
-
7. Perguntas com
- How are you?(Como você está?)

O pulque é uma bebida tradicional e fermentada feita a partir do suco extraído do agave, que tem sido consumida pelos povos indígenas do México há séculos. Com seu sabor único e levemente azedo, o pulque possui uma rica história ligada às antigas civilizações aztecas e toltecas, que já utilizavam o agave para produzir bebidas alcoólicas em cerimônias religiosas e celebrações.
A produção de pulque começa com a extração do "miel de agave" (mel de agave), que é fermentado naturalmente para produzir a bebida. Ao longo do tempo, o pulque passou a ser consumido de diversas formas, e, durante a era colonial, tornou-se uma bebida comum entre as classes populares do México, embora tenha perdido popularidade ao longo dos anos em favor de bebidas mais modernas, como a tequila e o mezcal.
O pulque era considerado uma bebida sagrada pelos aztecas e só poderia ser consumido por sacerdotes, guerreiros ou nobres. Sua popularidade se manteve, especialmente nas áreas rurais, e hoje é consumido principalmente no centro e sul do México, frequentemente servido em pulquerias, locais especializados na bebida.
Embora não seja tão comum como antes, o pulque continua sendo uma das bebidas mais representativas da cultura mexicana, conectando os mexicanos com suas raízes históricas e tradicionais.

O whisky canadense é amplamente reconhecido por sua suavidade e elegância, conquistando admiradores ao redor do mundo. Sua história remonta aos primeiros colonizadores do país, que trouxeram técnicas de destilação de whisky da Europa. No entanto, foi no Canadá que o whisky ganhou uma identidade própria, caracterizando-se por sua leveza e versatilidade, o que o torna uma bebida perfeita para ser apreciada pura, com gelo ou em coquetéis.
A produção de whisky no Canadá se espalhou pelas províncias, mas foi em Ontario e Quebec que as primeiras destilarias começaram a produzir esse destilado de maneira comercial, no século XIX. Ao longo do tempo, o Canadá se estabeleceu como um dos maiores produtores de whisky do mundo.
Marcas como Crown Royal, que foi criada em 1939 para celebrar a visita do Rei George VI e da Rainha Elizabeth ao Canadá, são exemplos de como o whisky canadense tem raízes profundas na história do país. Este whisky, em particular, é conhecido por sua mistura suave, composta principalmente de milho, o que lhe confere um sabor mais suave e doce do que outros tipos de whisky.
Hoje, o whisky canadense é uma bebida de prestígio, amplamente apreciada tanto em coquetéis como em sua forma pura, e continua a ser uma das bebidas alcoólicas mais representativas e respeitadas do país.

A Casa Rosada, sede do governo argentino, é um dos edifícios mais emblemáticos de Buenos Aires. Sua história remonta ao século XVI, quando o local abrigava um forte espanhol para proteger a cidade. Com o tempo, o edifício foi reformado e, no século XIX, sob o governo de Domingo Faustino Sarmiento, adquiriu sua icônica cor rosa, que simbolizaria a união entre os unitários (branco) e federalistas (vermelho), facções políticas rivais na época.
Localizada na Praça de Maio, a Casa Rosada foi palco de eventos históricos, como discursos de Juan e Eva Perón, além de manifestações políticas marcantes. Hoje, além de ser o centro do poder executivo, o local abriga o Museu da Casa Rosada, onde é possível conhecer objetos presidenciais, mobílias antigas e exposições sobre a história argentina.
Os visitantes podem fazer visitas guiadas gratuitas aos salões históricos, ao famoso balcão presidencial e explorar a rica arquitetura do palácio. Um passeio essencial para quem deseja entender a política e a cultura da Argentina.

Inaugurado em 1819, o Museu do Prado (Museo del Prado) é um dos maiores e mais prestigiados museus de arte do mundo. Localizado no coração de Madri, na Espanha, foi fundado pelo rei Fernando VII com o objetivo de exibir as coleções reais. O prédio neoclássico que o abriga foi projetado por Juan de Villanueva e mais tarde ampliado por outros arquitetos, como Rafael Moneo.
Com mais de 8.000 obras de arte, o museu é famoso por sua vasta coleção de pintura europeia, destacando-se por obras de mestres como Diego Velázquez, Francisco Goya, El Greco, Peter Paul Rubens e Hieronymus Bosch. Além das pinturas, o Prado possui esculturas, desenhos e artes decorativas.
Considerado o principal museu de Madri e um dos mais importantes da Europa, o Prado atrai milhões de visitantes anualmente. Sua impressionante coleção e sua contribuição para a preservação da arte clássica fazem do Museu do Prado uma parada obrigatória para qualquer amante da arte que visita a cidade.

O catalão é a língua oficial de Barcelona, e aprender algumas expressões pode enriquecer a experiência de qualquer turista. Aqui estão algumas frases úteis:
Bon dia – Bom dia
Bona tarda – Boa tarde
Bona nit – Boa noite
Com estàs? – Como você está?
Molt bé, gràcies – Muito bem, obrigado
On és el lavabo? – Onde é o banheiro?
Què tal? – Como vai?
Quant costa? – Quanto custa?
Tinc gana/sede – Estou com fome/sede
Un cafè si us plau – Um café, por favor
Perdona, on està...? – Desculpe, onde fica...?
Si us plau – Por favor
Gràcies – Obrigado
Adéu – Adeus
Em pots ajudar? – Você pode me ajudar?
M'agrada – Eu gosto
Embora muitos em Barcelona falem espanhol, usar o catalão é um sinal de respeito pela cultura local, e os barceloneses certamente apreciarão o esforço.

A gramática é o conjunto de regras e normas que governam o uso da linguagem. Ela define como as palavras são formadas, combinadas e organizadas para expressar ideias de maneira clara e eficiente. A gramática envolve aspectos como morfologia (estudo das palavras), sintaxe (ordenação das palavras nas frases), semântica (significado das palavras) e fonologia (sons da língua).
Estudar a gramática é essencial para dominar a língua, seja na comunicação escrita ou falada. Ao aprender as regras gramaticais, conseguimos entender e produzir textos de forma correta, coerente e precisa, além de evitar ambiguidades. A gramática também ajuda a preservar a identidade linguística e facilita a aprendizagem de outras línguas.
As primeiras tentativas de sistematizar a gramática surgiram na Antiguidade, com estudiosos como Platão e Aristóteles, mas foi com os romanos, como Cícero, que as primeiras regras mais estruturadas apareceram. A gramática moderna começou a ser formalizada por estudiosos do século XVI, como Port-Royal, na França. As regras gramaticais surgiram, então, do estudo e da observação das línguas ao longo do tempo, buscando padrões e normas para organizar o uso da linguagem.
A bioquímica é a ciência que estuda as reações químicas e processos moleculares que ocorrem nos organismos vivos. Essa área interdisciplinar combina princípios da química e da biologia para compreender o funcionamento das células, incluindo a estrutura e função de biomoléculas como proteínas, carboidratos, lipídios e ácidos nucleicos.
A bioquímica tem aplicações fundamentais na medicina, biotecnologia, nutrição e farmacologia, permitindo avanços na compreensão de doenças, no desenvolvimento de medicamentos e na engenharia de biomoléculas para diversos setores industriais. Técnicas como a engenharia genética e a biologia molecular impulsionam pesquisas sobre metabolismo, sinalização celular e regulação gênica.
Além disso, a bioquímica é essencial para áreas emergentes, como a bioquímica clínica e a biotecnologia industrial, que buscam soluções inovadoras para a saúde humana e sustentabilidade. Seu impacto se estende desde a descoberta de novos tratamentos até a produção de alimentos e biocombustíveis, tornando-se uma ciência indispensável para o avanço tecnológico e científico.

A série "A Grande Descoberta" se tornou um dos maiores sucessos da televisão, cativando milhões de espectadores com sua trama envolvente e cheia de mistério. Com uma média de 10 milhões de telespectadores por episódio, a produção rapidamente conquistou o topo das séries mais assistidas.
A história acompanha uma detetive experiente que se vê envolvida em um caso aparentemente simples de assassinato em uma pequena cidade. No entanto, conforme as investigações avançam, segredos profundos começam a ser revelados, transformando a investigação em uma teia de mistérios muito maior do que o esperado. A narrativa envolvente, aliada a atuações impactantes e uma cinematografia impecável, tornou a série um fenômeno de audiência.
Críticos elogiaram o roteiro bem estruturado e os personagens complexos, que garantem reviravoltas inesperadas a cada episódio. O sucesso foi tão grande que já há especulações sobre uma possível segunda temporada. "A Grande Descoberta" prova que o gênero de suspense policial continua sendo um dos favoritos do público, combinando tensão, drama e mistério em uma produção inesquecível.

Jurassic World: Renascimento chega às telonas como uma das maiores produções da franquia, mantendo a essência de ação e aventura que conquistou milhões de fãs ao redor do mundo. O filme, que faz parte da sequência da famosa série de dinossauros, traz uma nova e empolgante história, expandindo o universo de Jurassic World com novas ameaças, dinossauros nunca antes vistos e um enredo cheio de suspense.
Com uma trama que mistura nostalgia e inovação, o filme segue os personagens principais tentando controlar uma nova geração de dinossauros geneticamente modificados. Porém, a situação foge do controle, desencadeando um caos que coloca a humanidade em risco. O roteiro traz momentos de ação de tirar o fôlego e efeitos especiais de última geração, que são um grande atrativo para os fãs de ficção científica e cinema de entretenimento.
Jurassic World: Renascimento está fazendo sucesso em várias partes do mundo, quebrando recordes de bilheteiras e reafirmando o apelo universal da franquia. Com diretores e elenco de peso, o filme promete se tornar mais um marco na história do cinema, consolidando a popularidade das criaturas pré-históricas em uma nova geração de espectadores.
Em 2019, o mundo foi atingido por uma pandemia sem precedentes causada pelo coronavírus SARS-CoV-2, que levou à COVID-19, uma doença altamente contagiosa. O surto foi inicialmente detectado na China e rapidamente se espalhou pelo globo, resultando em milhões de infectados e mortes. Em março de 2020, a Organização Mundial da Saúde (OMS) declarou a COVID-19 uma pandemia.
O impacto na saúde pública foi devastador. Sistemas de saúde em vários países ficaram sobrecarregados, com hospitais lotados e falta de equipamentos essenciais. O vírus se espalhou rapidamente devido à transmissibilidade, obrigando governos a implementar medidas rigorosas de isolamento social e quarentenas.
Economicamente, a pandemia causou uma recessão global, com queda na produção, fechamento de empresas, aumento do desemprego e uma crise financeira sem precedentes. O comércio internacional também foi severamente afetado, e muitos países enfrentaram desafios para distribuir vacinas e tratamentos eficazes.
As interações sociais foram profundamente alteradas, com o distanciamento físico, o uso obrigatório de máscaras e a transição para o trabalho remoto e ensino online. A pandemia também destacou desigualdades sociais e econômicas, exacerbando problemas de saúde mental e bem-estar em todo o mundo.

Entre os séculos XVI e XVII, a Revolução Científica marcou uma mudança radical no conhecimento humano, desafiando crenças medievais e estabelecendo os fundamentos da ciência moderna. Grandes nomes como Nicolau Copérnico, Galileu Galilei e Isaac Newton revolucionaram a astronomia e a física, substituindo antigas concepções aristotélicas por métodos baseados na observação e na experimentação.
Em 1543, Copérnico publicou a teoria heliocêntrica, afirmando que a Terra girava em torno do Sol, contrariando a visão geocêntrica defendida pela Igreja. No século XVII, Galileu, com o uso do telescópio, confirmou essa teoria e desafiou a Inquisição, sendo julgado por heresia. Pouco depois, Newton formulou as leis do movimento e da gravidade, consolidando o método científico e a visão mecanicista do universo.
A Revolução Científica não apenas transformou a astronomia e a física, mas também influenciou a filosofia e a tecnologia, preparando o caminho para o Iluminismo e para os avanços da modernidade. Esse período redefiniu a relação entre ciência e religião e moldou o mundo contemporâneo, estabelecendo a razão como pilar do conhecimento humano.

Em 1453, a queda de Constantinopla pelos otomanos, liderados pelo sultão Maomé II, marcou o fim de um dos impérios mais antigos da história: o Império Bizantino. A conquista da cidade, um marco decisivo, alterou profundamente as dinâmicas políticas e comerciais da Europa.
Com o enfraquecimento do Império Bizantino, os otomanos estabeleceram seu domínio na região e passaram a controlar importantes rotas comerciais entre o Oriente e o Ocidente, impactando o comércio europeu. Isso levou as potências europeias a buscar novas rotas comerciais, estimulando a Era das Descobertas e as grandes viagens de exploração, como as de Cristóvão Colombo e Vasco da Gama, na tentativa de acessar as riquezas do Oriente.
Além disso, a queda de Constantinopla teve implicações culturais e intelectuais significativas. Muitos estudiosos bizantinos fugiram para o Ocidente, levando consigo manuscritos e conhecimentos que contribuíram para o Renascimento, um período de grande efervescência cultural, científica e artística na Europa.
Esse evento não apenas marcou o fim da Idade Média, mas também abriu caminho para a transição para a Idade Moderna, com grandes transformações no campo da política, cultura e economia.

O Homo floresiensis, popularmente conhecido como "o Hobbit", é uma das descobertas mais intrigantes no estudo da evolução humana. Essa espécie foi identificada em 2003, na ilha de Flores, na Indonésia, e surpreendeu a comunidade científica devido ao seu tamanho diminuto e características únicas, desafiando as concepções tradicionais sobre a evolução humana.
Com apenas cerca de 1 metro de altura e um crânio notavelmente pequeno, o Homo floresiensis viveu até cerca de 50 mil anos atrás, convivendo com humanos modernos. Sua descoberta gerou debates sobre as origens e evolução dos seres humanos, levantando questões sobre a possibilidade de que pequenos grupos de hominídeos sobreviveram por mais tempo do que se pensava.
Acredita-se que o Homo floresiensis tenha sido uma espécie isolada, provavelmente adaptada ao ambiente específico de Flores, onde o isolamento geográfico teria levado ao desenvolvimento de suas características físicas distintas, como o cérebro reduzido, que se aproximava em tamanho ao do Australopithecus, um ancestral mais antigo.
O Homo floresiensis desafia as narrativas convencionais da evolução humana, sugerindo que a história do nosso desenvolvimento pode ser mais complexa do que se pensava.

O esporte acompanha a humanidade desde os tempos mais remotos. Registros indicam que civilizações antigas, como egípcios e sumérios, já praticavam atividades físicas para lazer e treinamento militar. Na Grécia Antiga, os Jogos Olímpicos, iniciados em 776 a.C., consolidaram a competição esportiva, destacando provas como corrida e luta. Com a queda do Império Romano, os esportes perderam espaço, mas renasceram na Idade Moderna. No século XIX, países europeus padronizaram regras e criaram federações, impulsionando modalidades como futebol e atletismo. Hoje, o esporte é um fenômeno global, promovendo saúde, cultura e união entre nações.

Em 2021, Jujutsu Kaisen não apenas conquistou o público mundial com seu grande sucesso, mas também desempenhou um papel crucial na popularização do gênero shonen entre a geração mais jovem, de uma maneira inovadora. Uma curiosidade interessante que muitos não sabem é que o design do protagonista Yuji Itadori foi inspirado no próprio estilo do autor do mangá, Gege Akutami. O autor desenhou Yuji com características mais simples e "humanas", buscando criar uma conexão mais próxima com o público, facilitando a identificação com o personagem.
Além disso, Jujutsu Kaisen se destacou por uma colaboração inesperada com o mundo da moda. Diversas marcas de streetwear lançaram coleções inspiradas nos personagens e nas cenas icônicas da série, mostrando como a cultura do anime está cada vez mais influenciando outras indústrias, além da animação. A popularidade crescente do anime reflete a evolução de como a cultura pop japonesa está interagindo com a moda e outras áreas da sociedade contemporânea.
